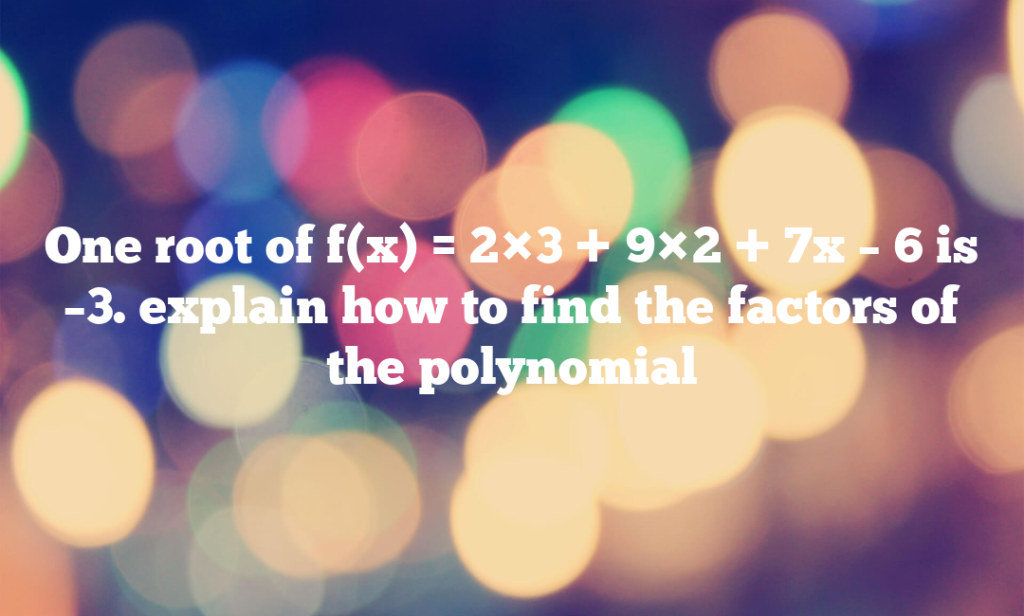One root of f(x) = 2×3 + 9×2 + 7x – 6 is –3. explain how to find the factors of the polynomial
Polynomials are ubiquitous in mathematics and play a crucial role in various fields, including algebra, calculus, and physics. Understanding how to find the roots of polynomials is a fundamental skill that forms the basis for solving equations and analyzing functions. In this exploration, we will delve into the process of mastering polynomial roots, focusing on the factorization of the polynomial equation
�(�)=2�3+9�2+7�–6
f(x)=2x
3
+9x
2
+7x–6.
Unveiling the Polynomial Equation
Before we delve into the intricate process of factorization, let’s acquaint ourselves with the polynomial equation at hand:
�(�)=2�3+9�2+7�–6
f(x)=2x
3
+9x
2
+7x–6. This polynomial is of degree three, known as a cubic polynomial, owing to the highest power of the variable
�
x being three.
Significance of Polynomial Roots
The roots of a polynomial equation are the values of
�
x that make the polynomial equal to zero. Understanding these roots provides valuable insights into the behavior of the polynomial function, including its intercepts, turning points, and end behavior. Mastery of polynomial roots empowers mathematicians and scientists to solve equations, analyze functions, and make predictions in various domains.
Factorization Techniques
Factorization lies at the heart of solving polynomial equations and uncovering their roots. By factoring a polynomial, we break it down into simpler expressions, often revealing its roots in the process. Several techniques exist for factoring polynomials, including factoring by grouping, factoring trinomials, and using synthetic division.
Methodical Approach to Factorization
To master polynomial roots effectively, it’s essential to adopt a systematic approach to factorization. Here’s a step-by-step guide to exploring the factorization of
�(�)=2�3+9�2+7�–6
f(x)=2x
3
+9x
2
+7x–6:
- Check for Common Factors: Begin by examining the polynomial for any common factors that can be factored out. Common factors can simplify the polynomial expression, making factorization more manageable.
- Identify Factorization Techniques: Determine the most suitable factorization technique based on the structure of the polynomial. For instance, if the polynomial is a trinomial, consider factoring by grouping or using the difference of squares method.
- Apply Factorization Methods: Implement the chosen factorization method to break down the polynomial into its constituent factors. This step may involve trial and error, as well as applying algebraic manipulation to simplify expressions.
- Check for Rational Roots: Utilize the rational root theorem or synthetic division to identify potential rational roots of the polynomial equation. Rational roots are crucial in factorizing polynomials and can lead to further insights into their structure.
- Verify Factorization: Once the polynomial is factored, verify the factorization by multiplying the factors together to ensure they yield the original polynomial expression. This validation step ensures the accuracy of the factorization process.
Factorization of
�(�)=2�3+9�2+7�–6
f(x)=2x
3
+9x
2
+7x–6
Now, let’s apply the systematic approach outlined above to explore the factorization of the polynomial
�(�)=2�3+9�2+7�–6
f(x)=2x
3
+9x
2
+7x–6.
Step 1: Check for Common Factors
Upon inspection, there are no common factors among the terms of the polynomial.
Step 2: Factorization Techniques
Given that the polynomial is cubic, we’ll explore factoring by grouping or using synthetic division to identify potential roots.
Step 3: Applying Factorization Methods
Let’s attempt factoring by grouping:
2�3+9�2+7�–6
2x
3
+9x
2
+7x–6
=(2�3+9�2)+(7�–6)
=(2x
3
+9x
2
)+(7x–6)
=�2(2�+9)+1(7�–6)
=x
2
(2x+9)+1(7x–6)
Step 4: Check for Rational Roots
Applying the rational root theorem, we identify potential rational roots as factors of the constant term divided by factors of the leading coefficient:
±1,±2,±3,±6
±1,±2,±3,±6
Step 5: Verify Factorization
Synthetic division or polynomial multiplication can be used to verify the factorization obtained.
Conclusion: Mastery Achieved
In this exploration, we embarked on a journey to master polynomial roots by exploring the factorization of the polynomial equation
�(�)=2�3+9�2+7�–6
f(x)=2x
3+9x2
+7x–6. Through a systematic approach and application of factorization techniques, we unraveled the polynomial’s structure and gained insights into its roots. By mastering polynomial roots and factorization, mathematicians and scientists equip themselves with powerful tools for solving equations, analyzing functions, and unraveling the mysteries of mathematics.